Chi-squared Distribution

Stats/Distribution 2020. 1. 31. 15:21

1. Overview

the chi-square distribution (also chi-squared or χ2-distribution) with k degrees of freedom is the distribution of a sum of the squares of k independent standard normal random variables. The chi-square distribution is a special case of the gamma distribution and is one of the most widely used probability distributions in inferential statistics, notably in hypothesis testing and in construction of confidence intervals. This distribution is sometimes called the central chi-square distribution, a special case of the more general noncentral chi-square distribution.

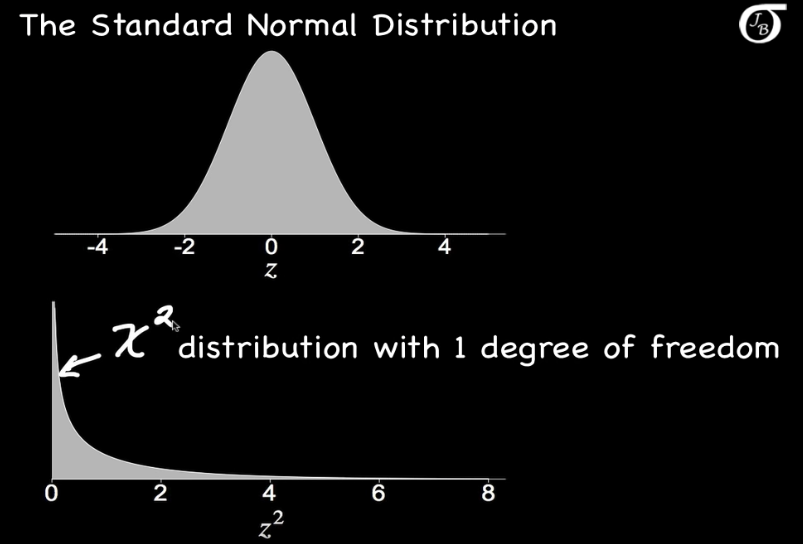
2. Chi-squared ($\chi ^{2}$) Distribution

$$Q\sim \chi ^{2}(k)=\sum_{i=1}^{k}Z_{i}^{2}=Z_{1}^{2}+Z_{2}^{2}+\cdots +Z_{k}^{2}$$

where k is degrees of freedom which is the number of $Z_{i}$s, each Zs are independent standard normal random variables.

Particular
Parameters	$k\in \mathbb{N}_{>0}$ (known as degrees of freedom)
Support	$x\in (0,+\infty )\: if\: k=1,otherwise\: x\in [0,+\infty )$
Mean	k
Median	$\approx k(1-\frac{2}{9k})^{3}$
Mode	max(k-2, 0)
Variance	2k
Skewness	$\sqrt{\frac{8}{k}}$
Excess kurtosis	$\frac{12}{k}$
Entropy	$\frac{k}{2}+ln(2\Gamma (\frac{k}{2}))+(1-\frac{k}{2})\psi (\frac{k}{2})$
Moment-generating function(MGF)	$(1-2t)^{-\frac{k}{2}}\: for\: t<\frac{1}{2}$
Characteristic function(CF)	$(1-2it)^{-\frac{k}{2}}$
Probability-generating function(PGF)	$(1-2ln\, t)^{-\frac{k}{2}}\: for\: 0<t<\sqrt{e}$

2.1 Probability density function (pdf) of $\chi ^{2}$ distribution

$$\frac{1}{2^{\frac{k}{2}}\Gamma (\frac{k}{2})}x^{\frac{k}{2}-1}e^{\frac{-x}{2}}\: for\: x\geq 0$$

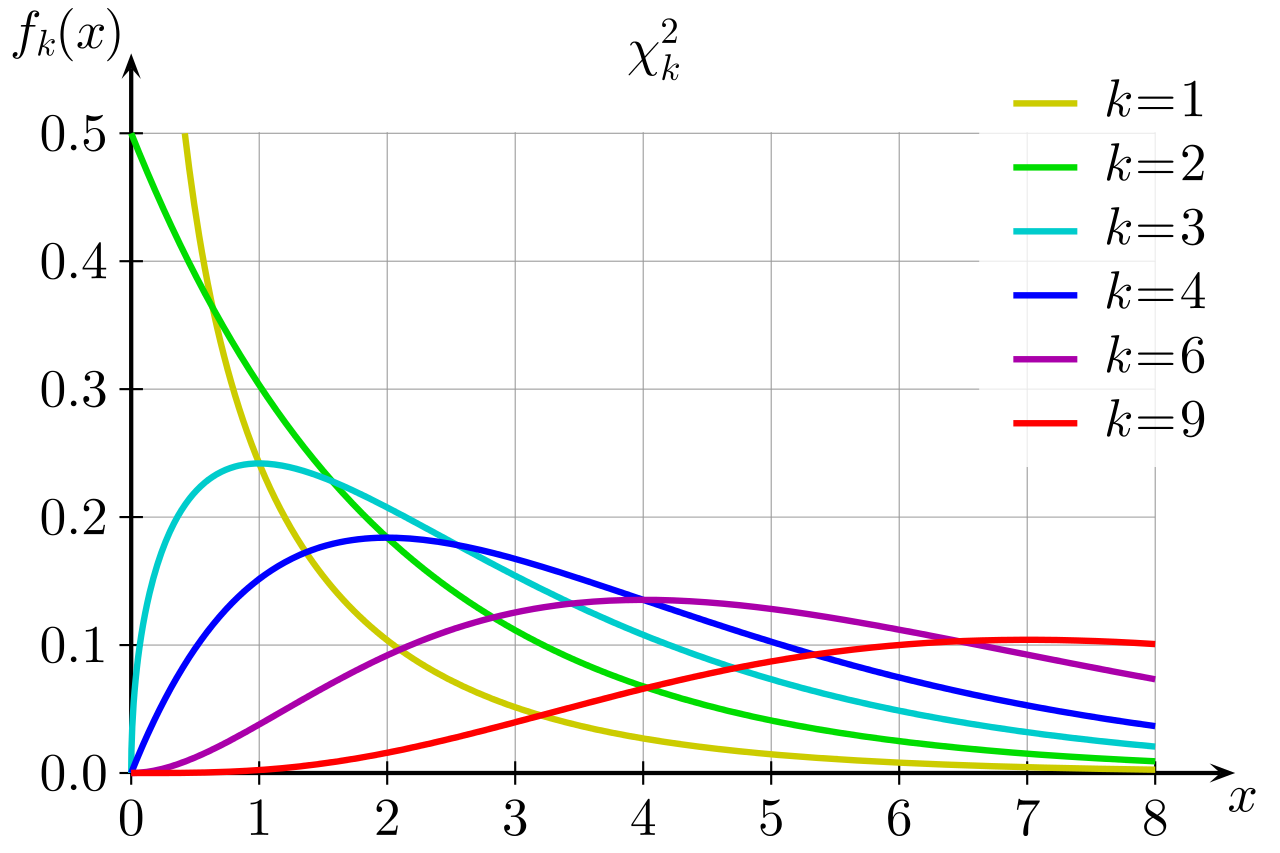
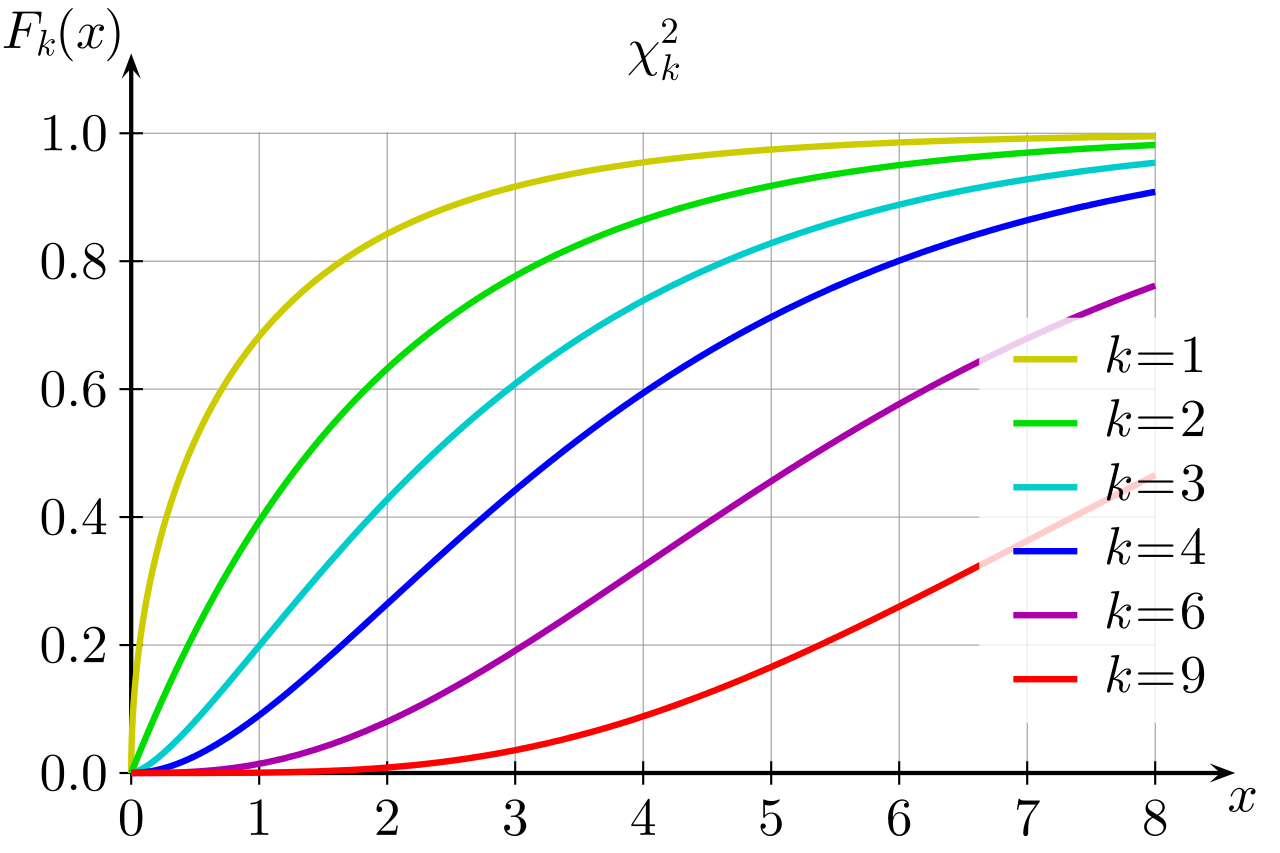
2.2 Cumulative distribution function (cdf) of $\chi ^{2}$ distribution

$$\frac{1}{\Gamma (k/2)}\gamma (\frac{k}{2},\frac{x}{2})$$

3. Table of $\chi ^{2}$ values vs p-values

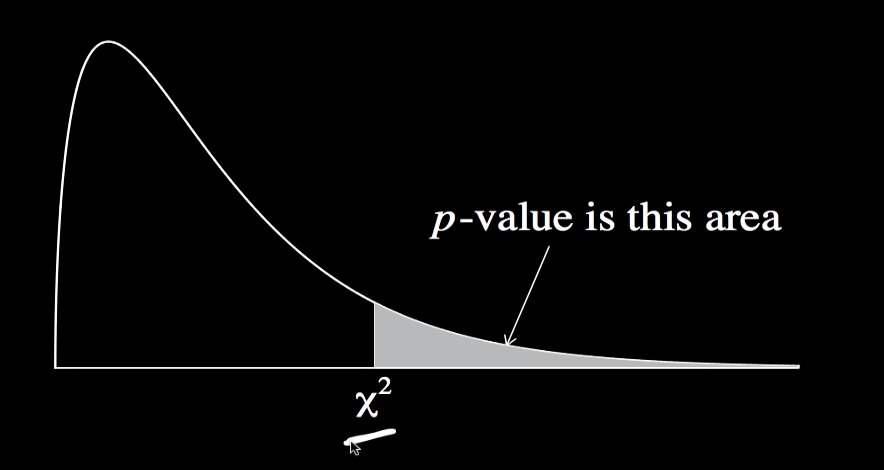
The p-value is the probability of observing a test statistic at least as extreme in a chi-square distribution. Accordingly, since the cumulative distribution function (CDF) for the appropriate degrees of freedom (df) gives the probability of having obtained a value less extreme than this point, subtracting the CDF value from 1 gives the p-value. A low p-value, below the chosen significance level, indicates statistical significance, i.e., sufficient evidence to reject the null hypothesis. A significance level of 0.05 is often used as the cutoff between significant and non-significant results.

The table below gives a number of p-values matching to $\chi ^{2}$ for the first 20 degrees of freedom and each significance level.

3.1 Left tailed distribution

3.2 Right tailed distribution

4. Example

4.1 If the value of the test statistic is 7.1, with 3 degrees of freedom, what is the p-value?

$$\chi_{a} ^{2}=\sum_{all\, cells}\frac{(Observed-Expected)^{2}}{Expected}$$

The degree of freedom is 3 and the critical value of statistic 7.1 falls into between 6.251 and 7.815. It means this value falls right to the significance level of 0.10 but the left of 0.05.

So p-value is

$$0.05<p-value<0.10$$