Quadratic form

 

1. Overview

all matrix is square

2. Description

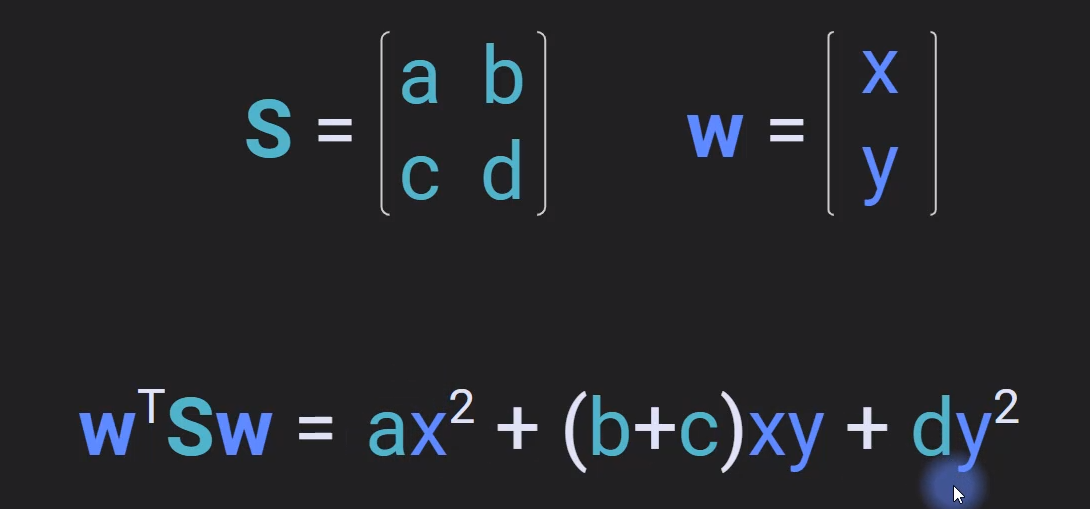
3. Example

What do they mean and how do you interpret them? So this is related to the concept of the energy in matrix S that I referred to.

If symmetric

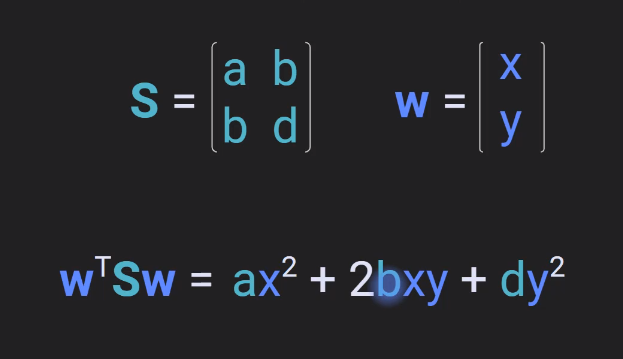
3 by 3 matrix

identity matrix

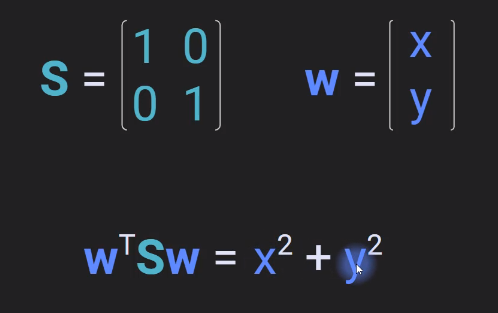
4. Quadratic form in geometry

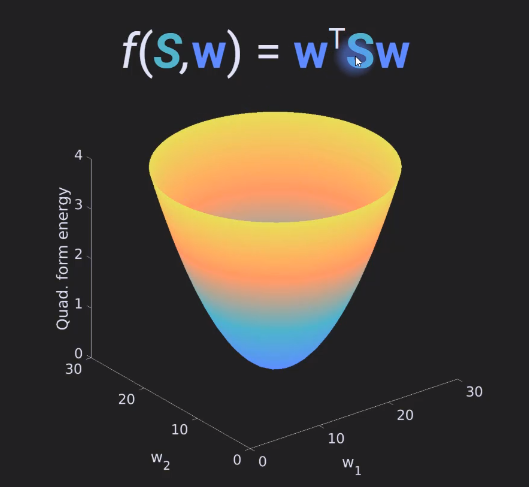
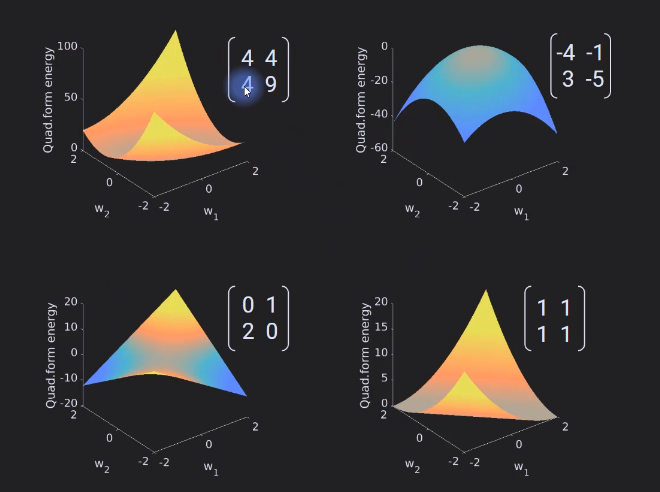
You should also note that all of these surfaces have a value of zero at the coordinate point W1 W2 both equals 0. And that's because when the vector W is the zero vector then of course the quadratic form works out to be 0.

5. Normalized quadratic form

now the question is which directions in this surface maximize the energy of the quadratic form for this particular matrix. Now for this specific matrix, the answer is every direction the energy of this matrix increases to infinity in every direction away from the origin. Maybe in some directions, the energy maximizes a bit faster but it's always increasing up to infinity.

There are interesting things to say about a matrix based on whether the quadratic form goes to plus infinity negative infinity or zero but it would also be nice for applications if we could have something like a quadratic form that tells us only about the Matrix and is not influenced by the vector elements going larger and larger or further away from zero. And that motivates including some kind of normalization factor that will allow us to explore the quadratic form in a way that's more independent of the vector w this idea is even more clear when you see that the matrix is the identity matrix.

What values of W and W2 or the elements in vector W will maximize the quadratic form? Well, obviously this expression goes to infinity as the values in w go to infinity and of course these values can be negative as well because you end up squaring the two terms. So for the case of the identity matrix this expression goes to infinity. When W goes to minus infinity or plus infinity and that is trivial and therefore the answer to this question how to maximize the energy in this particular quadratic form for the identity matrix it's trivial because the answer is driven by the vector and it doesn't really reflect the Matrix itself.

The solution is to normalize the quadratic form by the magnitude of W and that way we are learning something about the Matrix and normalizing out the trivial growth of the magnitude of the quadratic form resulting from increases in vector w.

So what we want is for this vector-only to provide a coordinate system for studying the energy landscape of this matrix. We don't actually care about the magnitude of this vector per se and that is why this is a useful normalization because the magnitude of the vector gets normalized out.

Okay, so this is called the normalized quadratic form. It's normalized for the magnitude of W and that was the main purpose of this video.

4. References